Identify The Ring Math . a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. If we take b = 1, then a = a, so that (b ) = ( b)a = ba. a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. Cancelling we get ab = ba. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: A + b = (a + b)2 b: We compute (a + b)2.
from www.youtube.com
A + b = (a + b)2 b: Cancelling we get ab = ba. We compute (a + b)2. a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: If we take b = 1, then a = a, so that (b ) = ( b)a = ba.
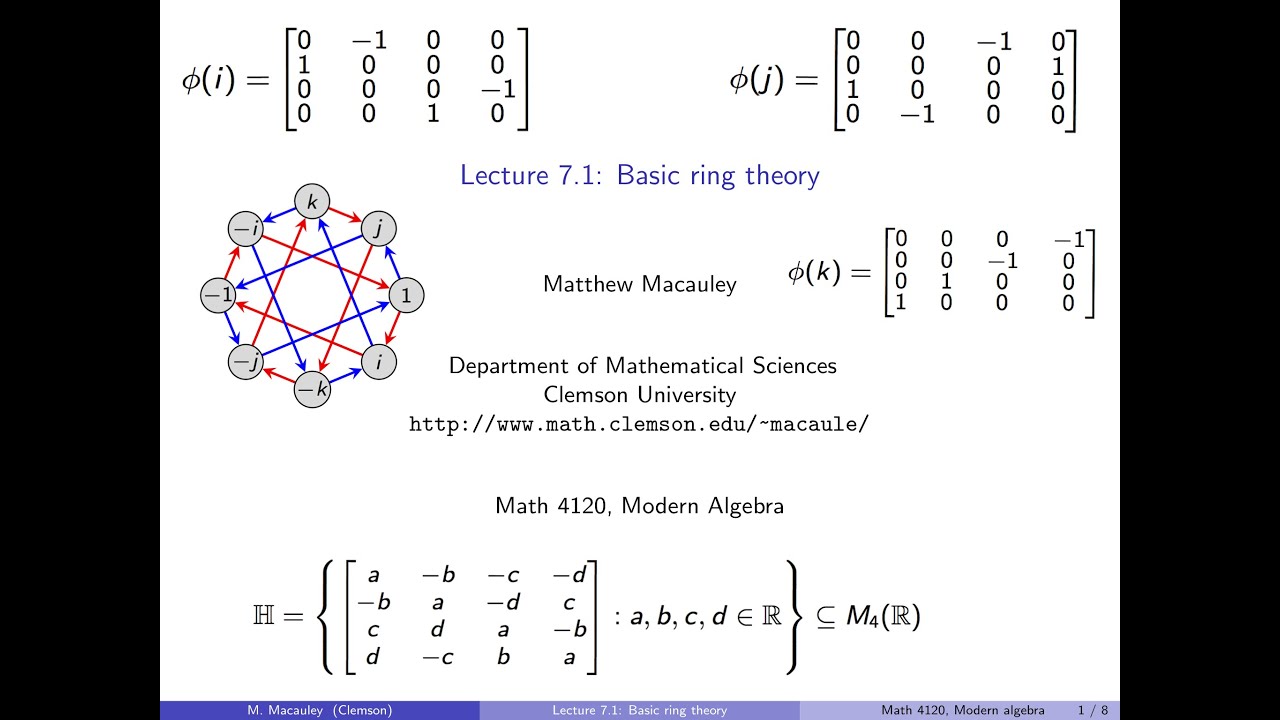
Visual Group Theory, Lecture 7.1 Basic ring theory YouTube
Identify The Ring Math A + b = (a + b)2 b: under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. Cancelling we get ab = ba. If we take b = 1, then a = a, so that (b ) = ( b)a = ba. any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. We compute (a + b)2. A + b = (a + b)2 b:
From www.pinterest.com
More Numbers in the Ring Numbers, Rings, Year three Identify The Ring Math any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: We compute (a + b)2. If we take b = 1, then a =. Identify The Ring Math.
From www.discountschoolsupply.com
Stack the Rings Identify The Ring Math a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. If we. Identify The Ring Math.
From donsteward.blogspot.com
MEDIAN Don Steward mathematics teaching olympic rings Identify The Ring Math any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. A + b = (a + b)2 b: a. Identify The Ring Math.
From flapjackeducation.com
Math Rings Game Identify The Ring Math a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. Cancelling we get ab = ba. any ring can be regarded as an algebra over the ring of. Identify The Ring Math.
From www.youtube.com
Ring Theory Commutative Ring Properties of Ring Example of Ring Identify The Ring Math under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: If we take b = 1, then a = a, so that (b ) = ( b)a = ba. Cancelling we get ab = ba. A + b = (a + b)2 b: any ring can be regarded as an algebra over. Identify The Ring Math.
From www.brainsyoga.com
Olympic Rings Math Brain Teaser for kids with Answer Identify The Ring Math A + b = (a + b)2 b: Cancelling we get ab = ba. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. a ring is a set equipped with two operations (usually referred to as addition. Identify The Ring Math.
From www.youtube.com
Abstract Algebra What is a ring? YouTube Identify The Ring Math A + b = (a + b)2 b: a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. We compute (a + b)2. Cancelling we get ab = ba. If we take b = 1, then a = a, so that (b ) = ( b)a = ba. under. Identify The Ring Math.
From www.youtube.com
Introduction to Higher Mathematics Lecture 17 Rings and Fields YouTube Identify The Ring Math a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. a ring in the mathematical sense is a. Identify The Ring Math.
From www.pinterest.ca
Equation Ring, Infinity Equation Ring, Math Ring, Math Jewelry, Science Identify The Ring Math a ring in the mathematical sense is a set s together with two binary operators + and * (commonly interpreted. a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. Cancelling we get ab = ba. A + b = (a + b)2 b: We compute (a + b)2.. Identify The Ring Math.
From www.youtube.com
B.SC. Sem (3); MATHS. Algebra; “ Ring Theory “ Theorem on Ring 01 Identify The Ring Math If we take b = 1, then a = a, so that (b ) = ( b)a = ba. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: Cancelling we get ab = ba. any ring can be regarded as an algebra over the ring of the integers by taking the. Identify The Ring Math.
From www.youtube.com
RINGS/prove that Q(√2)=(a+b√2) is a field under addition and Identify The Ring Math under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: A + b = (a + b)2 b: If we take b = 1, then a = a, so that (b ) = ( b)a = ba. a ring is a set equipped with two operations (usually referred to as addition and. Identify The Ring Math.
From www.youtube.com
L 31 Prime Ideal Ring Theory Ring Theory and Linear Algebra 1 B Identify The Ring Math any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. Cancelling we get ab = ba. We compute (a + b)2. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \. Identify The Ring Math.
From www.youtube.com
Abstract Algebra Types of rings. YouTube Identify The Ring Math Cancelling we get ab = ba. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: We compute (a + b)2. A. Identify The Ring Math.
From www.youtube.com
Visual Group Theory, Lecture 7.1 Basic ring theory YouTube Identify The Ring Math A + b = (a + b)2 b: If we take b = 1, then a = a, so that (b ) = ( b)a = ba. We compute (a + b)2. a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. Cancelling we get ab = ba. any. Identify The Ring Math.
From www.youtube.com
Abstract Algebra The definition of a Ring YouTube Identify The Ring Math If we take b = 1, then a = a, so that (b ) = ( b)a = ba. Cancelling we get ab = ba. A + b = (a + b)2 b: We compute (a + b)2. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and. Identify The Ring Math.
From www.youtube.com
RNT1.1. Definition of Ring YouTube Identify The Ring Math Cancelling we get ab = ba. If we take b = 1, then a = a, so that (b ) = ( b)a = ba. any ring can be regarded as an algebra over the ring of the integers by taking the product $ n a $ (where $ n $ is an. a ring is an ordered. Identify The Ring Math.
From www.youtube.com
Ring and its types lecture 49/discrete mathematics YouTube Identify The Ring Math under the ordinary operations of addition and multiplication, all of the familiar number systems are rings: a ring is a set equipped with two operations (usually referred to as addition and multiplication) that satisfy certain. A + b = (a + b)2 b: a ring in the mathematical sense is a set s together with two binary. Identify The Ring Math.
From donsteward.blogspot.com
MEDIAN Don Steward mathematics teaching olympic rings Identify The Ring Math Cancelling we get ab = ba. A + b = (a + b)2 b: We compute (a + b)2. a ring is an ordered triple \ ( (r, + ,\cdot)\) where \ (r\) is a set and \ (+\) and \ (\cdot\) are binary operations on \ (r\) satisfying. any ring can be regarded as an algebra over. Identify The Ring Math.